Are you a kindergarten teacher seeking creative ways and easy strategies that make a great math lesson? When teaching math in kindergarten, it’s so important to provide students with a variety of math strategies and tools they can use to solve addition and subtraction problems—and will then improve their math skills as a whole. By providing different strategies for students to choose from, this gives them a head start in being able to naturally differentiate math and hopefully make math fun for them. Once these math concepts are introduced to students, they will be able to choose from different strategies as their math skill levels change as needed, and then become better abstract math thinkers in the future, leaving them able to easily incorporate fast math into their daily routines.
Concrete Math Strategies
At the beginning of the year, kindergarten students have very concrete math thinking. Your lesson plans should include math strategies that require something physical for them to manipulate, real objects around the classroom (like wooden blocks), in order for them to make sense of math problems. For example, when it comes to a problem like 2 + 3, a great way to show your students this problem can look like setting out a group of two counting bears, and a group of three counting bears. Then students can count all the bears together to figure out the solution. The interactive aspect of these math lessons is a fun way to engage young students in their first year or two of elementary school.
Concrete math strategies for kindergarten look similar for a subtraction problem. For example, if you give students the problem 5-1, students can count out five cubes. Then, they remove one cube from their group and are able to count again to see the answer is four.
In these examples, students used counting bears and cubes as strategies to solve math problems. There are also many other various objects and tools they can use depending on what you have in your classroom. They can manipulate counters, rekenreks, and ten frames with moveable pieces to solve math problems—another excellent way to help children visualize their math. Even fingers are a great tool to use when students need those concrete math strategies in order to solve problems. It really all depends on your student since each gravitates toward different learning styles that are a more effective way to learn for them.
Transitional Math Strategies
As students become more comfortable with solving math problems, they begin transitioning towards more abstract thinking. They still need something to represent the problem, but now it doesn’t have to be something that they can touch and move. Instead, it can look more like drawings that can represent the tools they use.
For example, instead of using physical cubes to represent a problem, students may draw cubes. Rather than using a physical ten frame, students may draw a ten frame to help them solve a math problem. In a subtraction problem like 8-3, this would look like a student first drawing eight circles within a ten frame. Then, they can erase or cross out three of those circles to show that there are now only five left.
I think it is important to note that while we do want students to be able to draw these quicker pictures to solve problems, that probably won’t be the case in the beginning. As in, if you give students a word problem that involves four flowers plus six more flowers, you should fully expect to see 10 beautiful flowers drawn out on your students' paper!
Our transitional math thinkers can also use drawn strategies, such as number lines, tally marks, and even classroom tools like dominos and dice in their problem solving.
Abstract Math Strategies
When students move on to abstract math thinking, they are ready for more complex strategies. With these types of strategies, students can represent things with numbers rather than pictures or physical objects. Also, many of these strategies rely on using some mental math and prior math knowledge to solve problems so this is when they will pull from the best practices. from prior math activities.
For example, later in the year (and earlier in the year for some of our more advanced students) students can begin using strategies like double facts that they’ve memorized. When given a problem like 2+2, many students just know that 2+2=4 without having to give it much thought because their number recognition has improved.
Students at this stage might also be ready to use strategies like counting on from the first number in an addition problem, and counting backward from the beginning number in a subtraction problem. Students can even think about composing and decomposing numbers as a way to solve problems when they are at this stage, so they can see what smaller numbers come together to make up larger numbers.
Depending on what stage of math-thinking students are in, the math strategies that they can effectively use will be vastly different, but the basic math principles they've learned should still be a solid foundation for moving forward. That's why it’s so important for the best result, to present students with a variety of strategies that they can pull from as they need them, making them even better prepared for first grade and beyond. You can even keep the strategies you have taught on display using Math Strategies Posters. This way, students have a choice in what strategies they use, and math is naturally differentiated as students solve problems in their own different ways, and according to their own skill level.
You can find many of these strategies used in this 100th Day of School set too! Happy teaching!

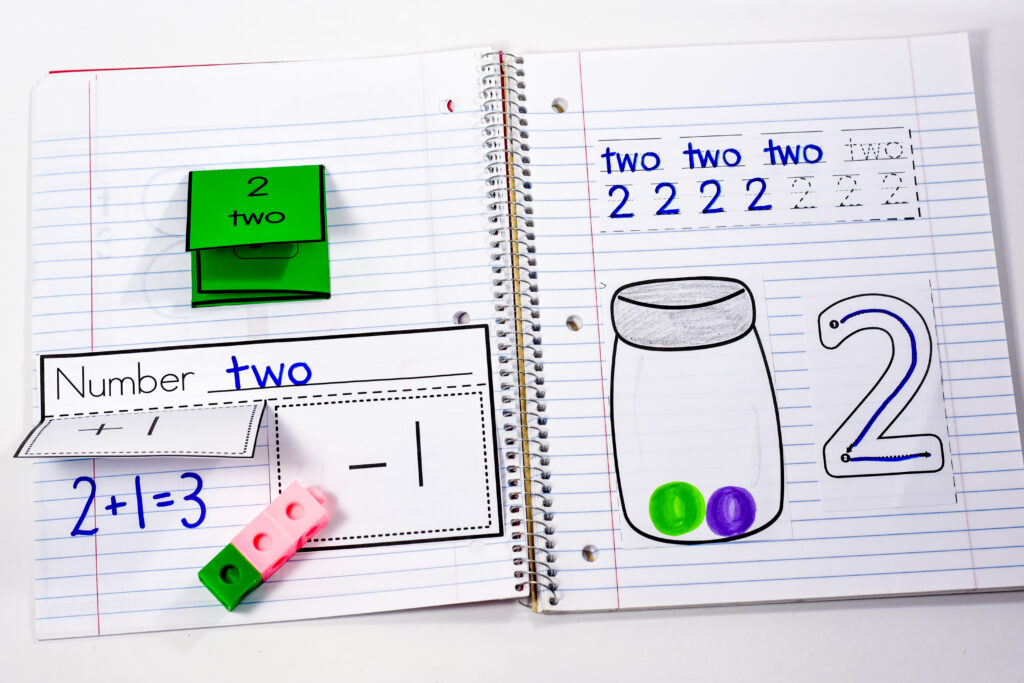










Leave a Reply